|
RKeff |
RKpsider |
 |
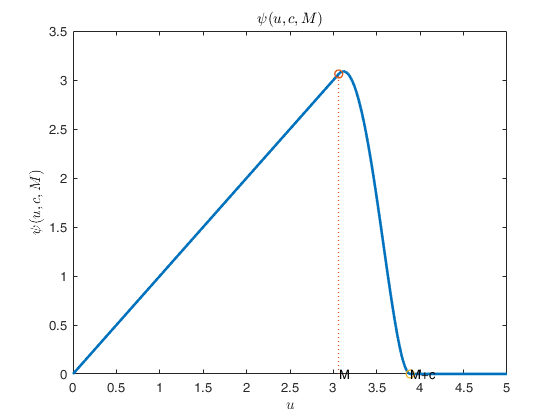
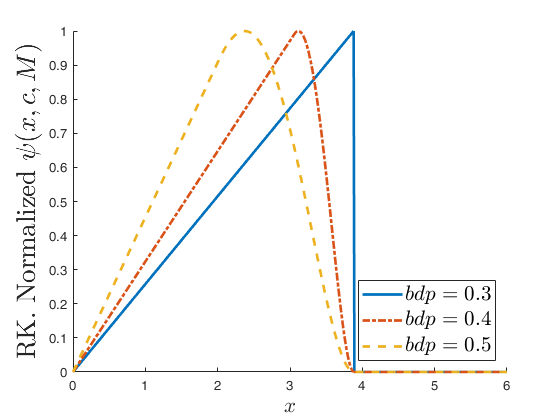
RKpsi
RKpsi computes psi function for Rocke (translated Tukey's) biweight
Syntax
psiRK=RKpsi(u,c, M)example
Examples
Plot of psi function.
Plot of psi function.
close all
% Find the values of c and M given bdp=0.4 and v=5 for ARP=0.01
x=0:0.01:5;
bdp=0.4;
v=5;
ARP=0.01;
[c,M]=RKbdp(bdp,v,ARP);
psiRK=RKpsi(x,c,M);
% psiRK=psiRK/max(psiRK);
plot(x,psiRK,'LineWidth',2)
xlabel('$u$','Interpreter','Latex')
ylabel('$\psi (u,c,M)$','Interpreter','Latex')
title('$\psi (u,c,M)$','Interpreter','Latex')
hold('on')
stem(M,M,'LineStyle',':','LineWidth',1)
text(M,0,'M')
stem(M+c,0,'LineStyle',':','LineWidth',1)
text(M+c,0,'M+c')
Related Examples
Compare Rocke psi function for 3 different values of bdp.
Compare Rocke psi function for 3 different values of bdp.
close all
x=0:0.01:6;
% Number of variables v is fixed to 5
v=5;
% ARP is fixed to 0.01
ARP=0.01;
lwd=2;
hold('on')
% Use bdp=0.3
bdp=0.3;
[c,M]=RKbdp(bdp,v,ARP);
psiRK030=RKpsi(x,c,M);
psiRK030=psiRK030/max(psiRK030);
plot(x,psiRK030,'LineStyle','-','LineWidth',lwd)
% Use bdp=0.4
bdp=0.4;
[c,M]=RKbdp(bdp,v,ARP);
psiRK040=RKpsi(x,c,M);
psiRK040=psiRK040/max(psiRK040);
plot(x,psiRK040,'LineStyle','-.','LineWidth',lwd)
% Use bdp=0.5
bdp=0.5;
[c,M]=RKbdp(bdp,v,ARP);
psiRK050=RKpsi(x,c,M);
psiRK050=psiRK050/max(psiRK050);
plot(x,psiRK050,'LineStyle','--','LineWidth',lwd)
xlabel('$x$','Interpreter','Latex','FontSize',16)
ylabel('RK. Normalized $\psi(x,c,M)$','Interpreter','Latex','FontSize',20)
legend({'$bdp=0.3$', '$bdp=0.4$' '$bdp=0.5$'},'Interpreter','Latex','Location','SouthEast','FontSize',16)
Input Arguments
Output Arguments
More About
References
Maronna, R.A., Martin D. and Yohai V.J. (2006), "Robust Statistics, Theory and Methods", Wiley, New York.
Rocke D.M. (1996), Robustness properties of S-estimators of multivariate location and shape in high dimension, "The Annals of Statistics", Vol. 24, pp. 1327-1345.
|
|
RKeff |
RKpsider |
|
 |
Functions |
|
• The developers of the toolbox • The forward search group • Terms of Use • Acknowledgments