vervaatrnd

vervaatrnd simulates random variates from the Vervaat perpetuity distribution.

expand all in page

Syntax

Description

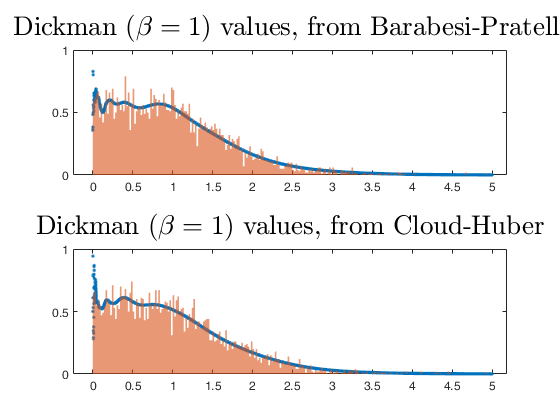
This function generates random numbers from a Vervaat perpetuity distribution with parameter $\beta \in (0,+\infty)$. Two alternative methods are implemented. The first method calls FSDA function vervaatsim, which is based on an accurate and efficient recursive simulation algorithm by Cloud and Huber (2018). The second method calls FSDA function vervaatxdf, which uses an algorithm by Barabesi and Pratelli (2019) to generate the pdf and cdf of the distribution. Below we give briefly some definitions, background, motivations and some key references.

example

X =vervaatrnd(betav) A random value from a Vervaat perpetuity with a default parameter.

example

X =vervaatrnd(betav, n) n random values from a Vervaat perpetuity with a specified betav parameter.

example

X =vervaatrnd(betav, n, method) n Vervaat perpetuity values extracterd with the two implemented methods.

Examples

expand all

  • A random value from a Vervaat perpetuity with a default parameter.

    • Argument betav is set to 1 (Dickman) if not specified. 

    clear all;
close all;
y=vervaatrnd

  • n random values from a Vervaat perpetuity with a specified betav parameter.

    • clear all;
close all;
n = 5;
betav = 2;
y=vervaatrnd(betav,5);

  • n Vervaat perpetuity values extracterd with the two implemented methods.

    • In the example, we set $n = 5000$ and $\beta = 1$. 

    % The superimposed normal kernel density is just for illustration: 
% a more precise density can be simulated with function vervaatxdf.
clear all;
close all
betav = 1;
N = 5000;
y1 = vervaatrnd(betav,N,1);
y2 = vervaatrnd(betav,N,2);
x    = (1:N)*(5*betav)/N;
pd1  = fitdist(y1(:),'Kernel','Kernel','normal','Support','positive');
pdf1 = pdf(pd1,x);
pd2  = fitdist(y2(:),'Kernel','Kernel','normal','Support','positive');
pdf2 = pdf(pd2,x);
figure;
h1 = subplot(2,1,1);
p1h = plot(x,pdf1,'.','LineWidth',2); ylim([0,1]);
if ~verLessThan('matlab','1.7.0')
hold on
h=histogram(y1);
h.Normalization='pdf';
h.BinWidth=0.02;
h.EdgeColor='none'; 
hold off
end
h2 = subplot(2,1,2);
plot(x,pdf2,'.','LineWidth',2); ylim([0,1]);
if ~verLessThan('matlab','1.7.0')
hold on
h=histogram(y2);
h.Normalization='pdf';
h.BinWidth=0.02;
h.EdgeColor='none'; 
hold off
end
title(h1,'Dickman ($$\beta = 1 $$) values, from Barabesi-Pratelli' ,'Fontsize',20,'interpreter','latex');
title(h2,'Dickman ($$\beta = 1 $$) values, from Cloud-Huber'       ,'Fontsize',20,'interpreter','latex');
    Click here for the graphical output of this example (link to Ro.S.A. website).

    Related Examples

    expand all

  • A time test for the two methods used to extract the Vervaat values.

    • Results can vary considerably with rep n and N (in this simulation method 1 computes the pascal matrix at each replicate). 

    clear all;
betav = 1;
rep = 10;
n = 10000;
N = randi(n,rep,1);
t1 = tic;
for i=1:rep;
y1 = vervaatrnd(betav,N(i),1);
end
t1end = toc(t1);
t2 = tic;
for i=1:rep;
y2 = vervaatrnd(betav,N(i),2);
end
t2end = toc(t2);
disp(['Barabesi-Pratelli: etime = ' num2str(t1end)]);
disp(['Cloud-Huber: etime = ' num2str(t2end)]);

  • N=5000 random values extracted from two Vervaat perpetuities.

    • Parameters are: $\beta = 1$ and $\beta = 10$. 

    % The superimposed normal kernel density is for illustration: 
% a more precise density can be simulated with function vervaatxdf.
close all
clear all;
close all;
betav10 = 10;
betav01 = 1;
N = 5000;
y10 = vervaatrnd(betav10,N);
y01 = vervaatrnd(betav01,N);
pdy10 = fitdist(y10(:),'Kernel','Kernel','normal','Support','positive');
x10   = (1:N)*(5*betav10)/N;
pdf10 = pdf(pdy10,x10);
pdy01 = fitdist(y01(:),'Kernel','Kernel','normal','Support','positive');
x01   = (1:N)*(5*betav01)/N;
pdf01 = pdf(pdy01,x01);
figure;
h1=subplot(2,1,1);
plot(x10,pdf10,'-','LineWidth',2); ylim([0,1]);
if ~verLessThan('matlab','1.7.0')
hold on
h=histogram(y10);
h.Normalization='pdf';
h.BinWidth=0.02;
h.EdgeColor='none'; 
hold off
end
h2=subplot(2,1,2);
plot(x01,pdf01,'-','LineWidth',2); ylim([0,1]);
if ~verLessThan('matlab','1.7.0')
hold on
h=histogram(y01);
h.Normalization='pdf';
h.BinWidth=0.02;
h.EdgeColor='none'; 
hold off
end
title(h1,'$$\beta = 10$$' ,'Fontsize',20,'interpreter','latex');
title(h2,'$$\beta = 1 $$ (Dickman)'  ,'Fontsize',20,'interpreter','latex');
    Click here for the graphical output of this example (link to Ro.S.A. website)

    Input Arguments

    expand all

    betav — Distribution parameter value. Positive integer.

    The parameter of the Vervaat family. Default is betav = 1.

    Data Types: single| double

    Optional Arguments

    n — Number of random numbers to extract. Positive integer.

    The sample size. Default is n = 1.

    Example: n=100.

    Data Types: Scalar.

    method — Computation method. Integer in {1,2} or structure.

    The method used to generate the random numbers: method = 1 (default) is for Barabesi and Pratelli (2019); method = 2 is for Cloud and Huber (2018). Method can be also a structure to pass the optional parameters for method 2, which are: - method.steps: Markov chain step. Positive integer. The inital number of steps to run to move the chain forward.

    Default is method.steps = 1.

    - method.d : Chain value at time 0. Positive integer or -1.

    The value of the dominating chain at time 0. Default is $D_0 \leftarrow x_0−1+G$, where $G \sim \mbox{Geo}(1/2)$ and $x_0 = \frac{1 + (2/3)^{1/\beta}}{1 - (2/3)^{1/\beta}}$ ($\mbox{Geo}$ represents the geometric distribution).

    Example: method = 2; Example - method = struct; method.steps=10; method.d=3;

    Data Types: Struct.

    Output Arguments

    expand all

    X —Random values extracted from the Vervaat perpetuity. Scalar or array

    The random value(s). Data Types - Double.

    More About

    expand all

    Additional Details

    A perpetuity is a random variable of the form: \[ Y = W_1 + W_1 \cdot W_2 + W_1 \cdot W_2 \cdot W_3 + \ldots \]

    where the $W_i$ are an independent, identically distributed (iid) sequence of random variables. If each $W_i$ has the same distribution, say $W_i \sim W$, then $Y \sim W(1 + Y)$ for $Y$ and $W$ independent.

    We are interested in this distribution because the running time of the Quickselect algorithm of Hoare, for finding the order statistics in a numerical array, approaches asymptotically a particular perpetuity, called Dickman distribution, with $W \sim Unif([0,1])$. Unfortunately such distribution has no closed form.

    The Dickman distribution can be also seen as a special case of Vervaat perpetuity, which is such that $W_i \sim U^{1/\beta}$ for some $\beta \in (0,\infty)$ for $U \sim Unif([0,1])$. In other words, the Dickman distributon is a Vervaat perpetutiy with $\beta = 1$.

    A generalization of the perpetuity takes the form

    \[ Y = \sum_{n=0}^{\infty}A_n \prod_{i}^{n} W_i\]

    with $A_n$ not necessarily equal to $1$, which is known as Takacs distribution.

    References

    Cloud, K. and Huber, M. (2018), Fast Perfect Simulation of Vervaat Perpetuities, "Journal of Complexity", Vol. 42, pp. 19-30.

    Devroye, L. (2001), Simulating perpetuities, "Methodology And Computing In Applied Probability", Vol. 3, Num. 1, pp. 97-115.

    Fill, J. A. and Huber, M. (2010), Perfect simulation of Vervaat perpetuities, "Electronic Journal of Probability", Vol. 15, pp. 96-109.

    Devroye, L. and Fawzi, O. (2010), Simulating the Dickman distribution, "Statistics and Probability Letters", Vol. 80, pp. 242-247.

    Blanchet, J. H. and Sigman, K. (2011), On exact sampling of stochastic perpetuities, "Journal of Applied Probability", Vol. 48A, pp. 165-182.

    Takacs, L. (1955), On stochastic processes connected with certain physical recording apparatuses. "Acta Mathematica Academiae Scientificarum Hungarica", Vol. 6, pp 363-379.

    Barabesi, L. and Pratelli, L. (2019), On the properties of a Takacs distribution, "Statistics and Probability Letters", Vol. 148, pp. 66-73.

    See Also

    | |

    This page has been automatically generated by our routine publishFS